Triangle With Sides 3 4 5 Angles
A triangle has one side length of 8cm and an adjacent angle of 45.5.
Triangle with sides 3 4 5 angles. Slant height = 5 cm. If the sides of a triangle are produced in an order, show that the sum of the exterior angles so formed is 360°. Rule 4 Remote.
The ratio 30 to 40 to 50 is equivalent to 3-4-5, and thus side AB is 50 units long. If you can "find" this triangle in your corner, you know the corner is square. Find the volume and surface area of the double cone so formed.
A triangle or trigon is a two dimensional geometric object that has the specific qualities of having three straight sides that intersect at three vertices. When you are given the lengths of two sides of a right triangle, check the ratio of the lengths to see if it fits the 3:4:5 ratio. A a triangle with angles of 30°, 60°, and 90° B an angle of 90° C a triangle with sides of 6, 8, and 10 D a triangle with sides of 3 and 4 E a triangle with a side measuring 4, next an angle of 90°, and next a side measuring 3 F a triangle with a side measuring 3, next an angle of 60°, and next a side measuring 4.
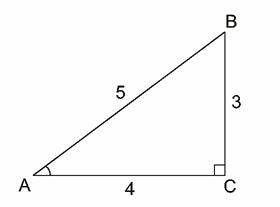
Therefore, side AB represents the 5-unit side of the triangle. A right triangle with sides of 3,4, and 5 units has angles that are 37 degrees, 53 degrees, and 90 degrees, respectively. Side a = 3 side b = 5 side c = 7 Since you know all 3 sides, then you can use the law of cosines to find one of the angles.
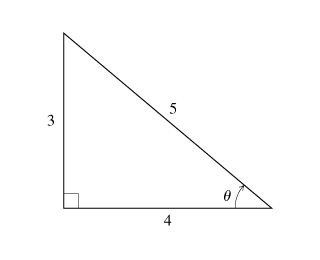
Sides "a" and "b" are the perpendicular sides and side "c" is the hypothenuse. Our leg a is 10 ft long, and the α angle between ladder and ground equals 75.5°. The other two are approximately 36.87° and 53.13°.
And a scalene triangle is a triangle where none of the sides are equal. Sin (90 degrees) = 1 so in the sin rule we have:. Pythagoras Theorem states that in a right angled triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.
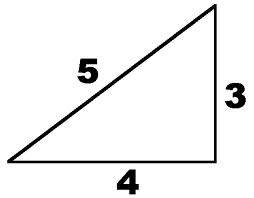
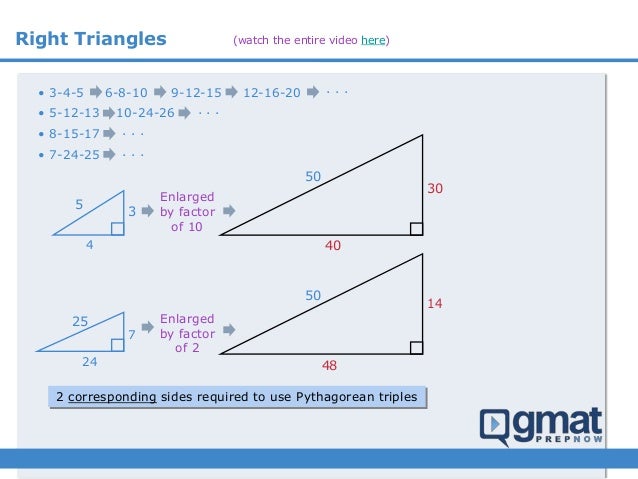
In this situation, 3, 4, and 5 are a Pythagorean triple. 5, or of other special numbers such as the golden ratio. A Pythagorean triple is a right triangle where all the sides are integers.
Since these sides are in the ratio 3 to 4 and angle C is 90°) the triangle is a 3-4-5 triangle. If the triangle is ABC we have angles A, B and C and sides AB, BC and CA. The rule says that:.
This is called an "angle based" right triangle. Calculation of the given by the length of three sides:. We have to use the sine rule here.
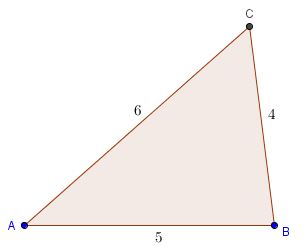
For example, if the sides are 3 in, 4 in, and 5 in, then the perimeter is simply 3 + 4 + 5 = 12 inches in total. Using a compass, from one vertex draw an arc with a 3 cm radius. The sum of the internal angles that exist at the vertices always total the same number for every triangle — 180 degrees, or radians.
Relationship between measurement of the sides and angles in a triangle:. It follows that any triangle in which the sides satisfy this condition is a right triangle. A "side-based" right triangle is one in which the lengths of the sides form ratios of whole numbers, such as 3 :.
It will even tell you if more than 1 triangle can be created. Solve problems with 3-4-5 right triangles. Computed angles, perimeter, medians, heights, centroid, inradius and other properties of this triangle.
Interior Angles Because it is a right triangle one angle is obviously 90°. Volume of cone = = = Total surface area of cone =. From the other vertex draw an arc with a 4 cm radius.
And - you guessed it - one of the most popular Pythagorean triples is the 3-4-5 right triangle. Use The Law of Cosines to find angle X first:. You could also use the Sum of Angles Rule to find the final angle once you know 2 of them.
A 3-4-5 triangle is right triangle whose lengths are in the ratio of 3:4:5. The largest interior angle and side are opposite each other. Let measures of the given angles of a triangle be 2x, 3x and 5x.
AB/sin (C) = BC/sin (A) = CA/sin (B) In a 3:4:5 triangle = AB:BC:CA we know CA = 5 is the hypotenuse and its opposite angle B is 90 degrees. Draw a triangle, say #A, B, C# with the given magnitudes. Knowing the ratios of the angles or sides of these special right triangles allows one to quickly calculate various lengths in geometric problems without resorting to more advanced methods.
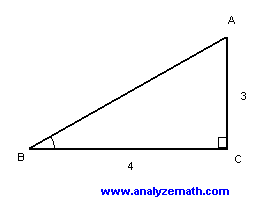
Area of a triangle with fixed perimeter;. To be congruent the triangles have to be the same size with the same angles. (Choose value of as found appropriate.) Let ABC be the right triangle where AB = 3cm and BC = 4 cm Since AC is the hypotenuse AC2 = AB2 + BC2 AC2 = 32 + 42 AC2.
This can be checked with the "Law Of Sines":. The area of a triangle with sides 3, 4 and 5 has to be determined using two methods. Calculate c = √a² + b² - 2ab * cos(γ).
It is seen that 3^2 + 4^2 = 9 + 16 = 25 = 5^2 The given triangle is a right-angled triangle. Math Warehouse's popular online triangle calculator:. Draw an arc 400 away from the start of the 300 line.
This is called an "angle-based" right triangle. This tool is designed to find the sides, angles, area and perimeter of any right triangle if you input any 3 fields (any 3 combination between sides and angles) of the 5 sides and angles available in the form. A triangle is a geometrical object that has three angles, hence the name tri–angle.Any valid plane triangle must adhere to the following two rules:.
Cos X = ((7.9) 2 + (3.5) 2 − (5.1) 2)/(2×7.9×3.5) cos X = (62.41 + 12.25 − 26.01)/55.3. Understand the 3-4-5 method. Knowing the ratios of the angles or sides of these special right triangles allows one to quickly calculate various lengths in geometric problems without resorting to more advanced methods.
Let ABC be a right triangle with sides = 3 inches, = 4 inches, and = 5 inches. Draw an arc 500 away from the end of the 300 line. Let the triangle be ABC with side a opposite angle A, side b opposite angle B, side c opposite angle C.
Ladder length, which is our right triangle hypotenuse, appears!. We are given that A right angle triangle ABC with sides 3 cm, 4 cm, 5 cm is revolved about the fixed side of 4 cm. If we know two of the side lengths and they are congruent with the 3 4 5 ratio, we can easily determine the third side length by using the ratio.
This rule says that if one side of a triangle measures 3 and the adjacent side measures 4, then the diagonal between those two points must measure 5 in order for it to be a right triangle. A "side based" right triangle is one in which the lengths of the sides form a whole number ratio, such as 3-4-5. Draw a 300 line along the wall.
Use The Law of Cosines to solve for the angles. Sum of Angles in a Triangle. Which of its sides is the hypotenuse?.
If a triangle has sides measuring 3, 4, and 5 feet (or any other unit), it must be a right triangle with a 90º angle between the short sides. Enter side a, side b and side c and click the button "Calculate the area of a triangle", Area of a triangle is displayed is calculated from the length of the three sides. Connect the three vertices with straight lines.
One of the two most famous is the 3–4–5 right triangle, where 3 2 + 4 2 = 5 2. Which side is opposite of the 53 degree angle?. Three sided polygon is called equilateral.
A 3 4 5 triangle is an SSS right triangle (meaning we know the three side lengths). Given two triangle sides and one angle;. For example 6, 8, and 10.
If you multiply the sides by any number, the result will still be a right triangle whose sides are in the ratio 3:4:5. It is a common Pythagorean triple that is worth memorizing to save time when dealing with right triangles. For example, a right triangle may have angles that form simple relationships, such as 45°–45°–90°.
So for example, if I have a triangle like this, where this side has length 3, this side has length 4, and this side has length 5, then this is going to be a scalene triangle. The angle β = 14.5° and leg b = 2.586 ft are displayed as well. Find the perimeter of a triangle.
We are given three sides of a triangle #3, 4 and 6#. (1) the sum of two sides of a triangle must be greater than the third side, and (2) the sum of the angles of a plane triangle must be equal to 180°. The same rule applies to the smallest sized angle and side, and the middle sized angle and side.
Triangle 4 4 5. How does this right triangle calculator work?. Enter the length of any two sides and leave the side to be calculated blank.
Cos X = (y 2 + z 2 − x 2)/2yz. In Degrees A + B + C = 180° In Radians A + B + C = π. If the angle is between the given sides, you can directly use the law of cosines to find the unknown third side, and then use the formulas above to find the missing angles, e.g.
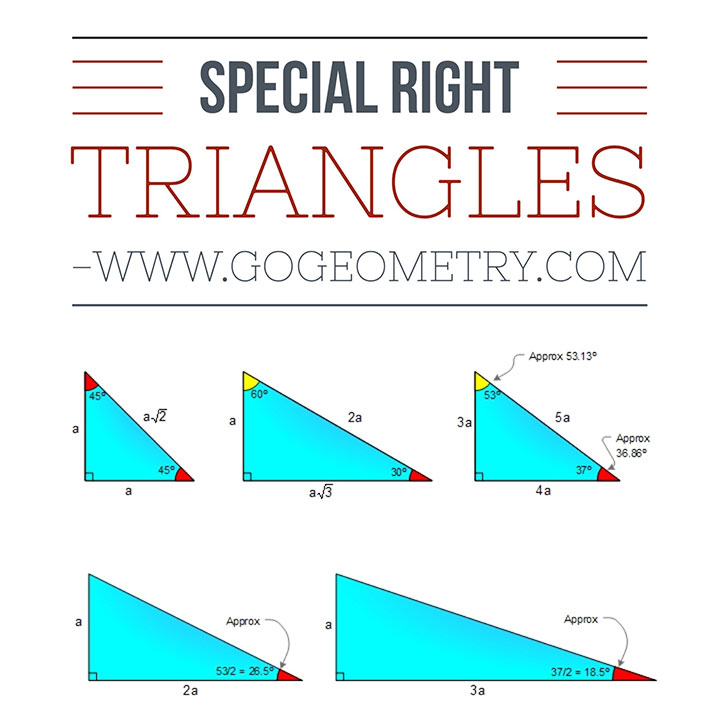
3-4-5 (The lengths of the sides form a whole number ratio), approx angles 37-53. A right triangle has two sides perpendicular to each other. Enter any valid combination of sides/angles(3 sides, 2 sides and an angle or 2 angle and a 1 side) , and our calculator will do the rest!.
The side opposite the 90° angle is always the hypotenuse. A triangle has angles of. The other common SSS special right triangle is the 5 12 13 triangle.
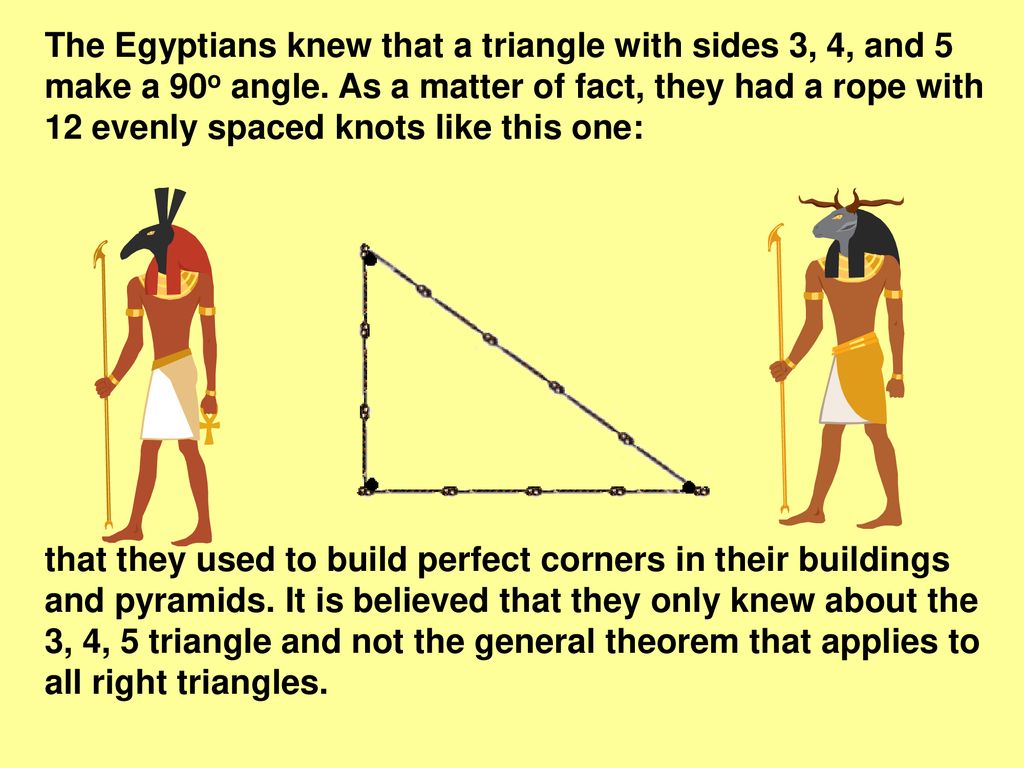
Connect from the start of the 300 line to where the arcs cross. The 3:4:5 triangle is the best way I know to determine with absolutely certainty that an angle is 90 degrees. The 3 side is opposite the 36°52'11.6315" angle and the 4 side is opposite the 53°07'48.3685" angle.
The beauty and simplicity of this technique are if the carpenter or builder needs to increase accuracy on larger walls or structures, any multiple of the 3-4-5 rule can be deployed. You decide to use 300, 400 and 500 cm lines. To determine whether the three given sides form a right triangle, we use the Pythagoras Theorem to verify.
This is based on the Pythagorean Theorem from geometry:. There are also special cases of right triangles, such as the 30° 60° 90, 45° 45° 90°, and 3 4 5 right triangles that facilitate calculations. Draw a straight line 6 cm in length.
If the sides of a triangle are $4,5,6$ prove that the largest angle is exactly double the smallest angle. Area of an equilateral triangle;. 3 and 4 are the lengths.
A 2 + B 2 = C 2 for a right triangle. The angles of a triangle are in the ratio 2:3:5. The other common SSS special right triangle is the 3 4 5 triangle.
The second leg is also an important parameter, as it tells you how far the ladder should be removed from the. Please check out also the Regular Triangle Calculator and the Irregular Triangle Calculator. If so, I'll ignore the fact that a triangle with sides 3, 4, and 5 would have angles of 37, 53 and 90 not 30, 60 , 90, because that's not what they are trying to assess.
Which side is opposite the 37 degree angle?. The 5 12 13 triangle is an SSS special right triangle with the ratio between its side lengths as 5, 12, and 13. 7^2 = 3^2 + 5^2 - 2*3*5*cos(C) Simplify to get:.
Knowing the relationships of the angles or ratios of sides of these special right triangles allows one to quickly calculate various lengths in geometric problems. C^2 = a^2 + b^2 - 2*a*b*cos(C) This becomes:. If the side opposite the angle is , what is the length of the side opposite ?.
In Euclidean geometry, any three non-collinear points determine a unique triangle and a unique plane. According to the cosine rule (also known as the Law of Cosines), the angle math\theta/math opposite the longest side satisfies the following equation:. Each end of the line is a vertex of the triangle.
Triangles that do not have an angle measuring 90° are called oblique triangles. Ex 13.5, 2 A right triangle, whose sides are 3 cm and 4 cm (other than hypotenuse) is made to revolve about its hypotenuse. Any triangle with sides of 3, 4, and 5 feet will have a 90-degree angle opposite the 5-foot side.
The other one is an isosceles triangle that has 2 angles measuring 45 degrees (45–45–90 triangle). The three sides of an equilateral triangle measure x+ 12, 2x- 5 and 3 x- 22in. An exterior angle of a triangle is equal to the sum of the two remote interior angles.
Your answer of A for Q.13:. Area by the "side angle side" method;. Without reference to tables or to the rule of Pythagoras, solve the following.
It's equal to 10.33 ft. The point where the two arcs intersect is the third vertex of the triangle. In this triangle we know the three sides x = 5.1, y = 7.9 and z = 3.5.
None of the sides have an equal length. 4 4 5 triangle. Area of a triangle whose side a is 3, side b is 4, and side c is 5.
First of all, that triangle won't work. If the area of the triangle is 18.54cm, calculate the length of the other side that encloses the 45.5 angle Thanks Eugene Brennan (author) from Ireland on May 13, :. 3Calculate the area of a triangle using the formula from the length of the sides.
Heron's formula works equally well in all cases and types of triangles. 4 4 5 - Acute isosceles triangle, area=7.81. The pattern for is that the sides will be.
And you have your "3,4,5" triangle with its right angle. A 3-4-5 triangle has angles of 90°, 36°52'11.6315" and 53°07'48.3685" approximately. So, height of the cone so formed is 4 cm.
In the simplest scenario one has measured all three sides of a triangle and then it is a matter of simple summation to find the perimeter. Where a and b are two sides of a triangle, and c is the hypotenuse, the Pythagorean theorem can be written as:. Side based right triangle:.
Given the sizes of the 3 sides you can calculate the sizes of all 3 angles in the triangle. The sides of the triangle measure. There is no need to calculate angles or other distances in the triangle first.
Area of a triangle;.
Pythagorean Theory Ppt Download

Answered A The Interior Angles Of A Triangle Bartleby

How To Find The Length Of The Hypotenuse Of A Right Triangle Pythagorean Theorem Sat Math
Triangle With Sides 3 4 5 Angles のギャラリー
Ex 11 1 6 Draw A Triangle Abc With Side 7 Cm B 45 A 105

Calameo Congruence Booklet
3 4 5 Rule Youtube
Act Maths Practice Questions With Detailed Solutions Sample 1

If The 3 Sides Of A Right Angle Triangle Are In Ap Then Prove That They Are In The Ratio 3 4 5 Brainly In

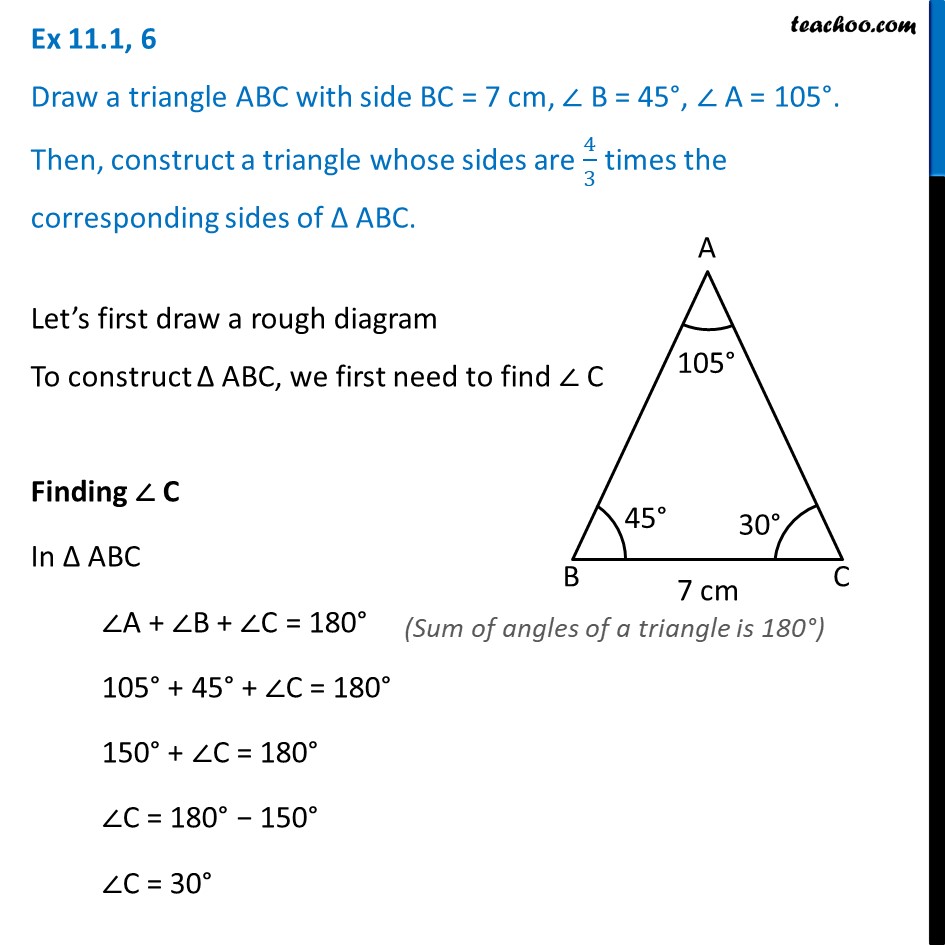
Draw A Right Triangle Which The Sides Other Than Hypotenuse Are Of Lengths 4 Cm And 3 Cm Then Construct Another Triangle Whose Sides Are 53 Times The Corresponding Sides Of The Given Triangle

Resources 3 4 5 Principle

Triangle Inequality Theorem To Use With Straws Triangle Inequality Inequality Kids Math Worksheets

Solved The 3 4 5 Right Triangle As We Noted In Section 6 Chegg Com

3 4 5 Triangle

Pythagorus Theorum Math Lesson 3 4 5 Triangle Youtube

Laying Out An Accurate Archery Range Using Stem Principles

3 4 5 Triangle

Calculating Angles For A 5 12 13 Triangle Video Lesson Transcript Study Com
Solution I Don 39 T Understand This Problem Please Help Which Set Of Integers Cannot Be Lengths Of The Sides Of A Triangle 1 3 4 5 2 3 4 4 3 2 3 4 4 3 1 1
What Is The Value Of Cos 53 Quora
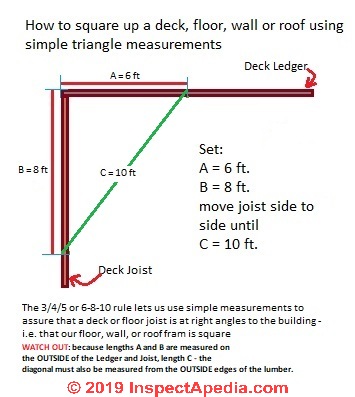
Use Triangles Simple Geometry To Aid Building Framing
Q Tbn 3aand9gcsfbhiwu8nl Wesbiylrrwtmvuq4m5fvqm0ac75zmy Fkyfvcjn Usqp Cau
Area Of Equilateral Triangle Video Khan Academy

Angle Geometry Of Pyramids Gary Osborn
The Sides Of A Triangle Are In The Ratio 3 4 5 The Measure Of The Largest Angle Of The Triangle Youtube

Ratios In Right Triangles Ppt Video Online Download

Special Right Triangles Solutions Examples Videos

If The Lengths Of The Sides Of A Triangle Are In A P And The Grea
Q Tbn 3aand9gcqa54uzitgrykbapp Ka1erwxxqb1l6ohi7qlhb2mn5 T4wb6ja Usqp Cau
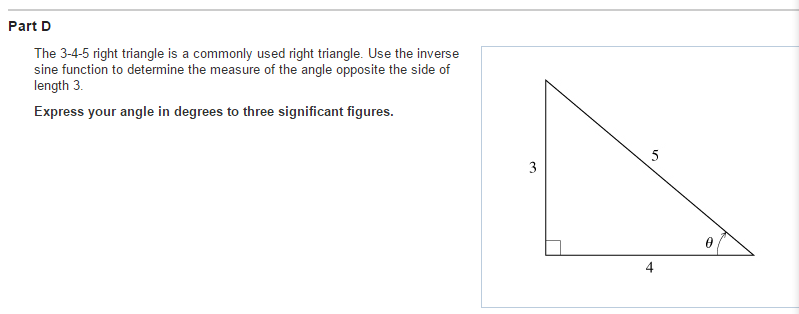
Solved Part Dthe 3 4 5 Right Triangle Is A Commonly Used Chegg Com

Village Level Brickmaking Appendices Appendix 3 The 3 4 5 Method
Http Www Foresthillshs Org Ourpages Auto 18 2 12 Geom similar triangle rules Pdf

5 12 13 Triangle Angles Sides How To Solve Full Lesson

Three Equal Charges Each Having A Magnitude Of 2 0 10 6 C Are Placed At The Three Corners Of A Right Angled Triangle Of Sides 3 Cm 4 Cm And 5 Cm

Right Triangle Calculator Definition Formula

Special Right Triangle Wikipedia

The Length Of The Sides Of A Triangle Are In The Ratio 3 4 5 And Its Perimeter Is 144 Cm Find The Brainly In
Angles In 4 5 6 Triangle
1
What Is The Length Of The Side Of The Largest Square Which Is Cut Out From A Right Angled Triangle Of Lengths 3 4 5 Cms In Such A Way That One

Printable
Properties Of Triangle Angle Properties Of Triangles Definition Of Triangle
Q Tbn 3aand9gcrfviva8aik6sfaebzrvu1sb760sjxfcy8idaurb9fj73mcr Uo Usqp Cau

Calculating Angles For A 5 12 13 Triangle Video Lesson Transcript Study Com
How To Solve Problems With The Altitude 0n Hypotenuse Theorem Dummies

Phy10 Brief Trigonometry Tutorial Accessible Physics Concepts For Blind Students Openstax Cnx
The 3 4 5 Method For Squaring Corners Concord Carpenter
Www Brewtoncityschools Org Cms Lib Al Centricity Domain 133 5 5 The Triangle Inequality Pdf
What Is The Value Of Cos 53 Quora
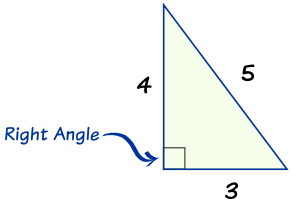
Math Year 13 The 3 4 5 Triangle

Properties Of 3 4 5 Triangles Definition And Uses Video Lesson Transcript Study Com
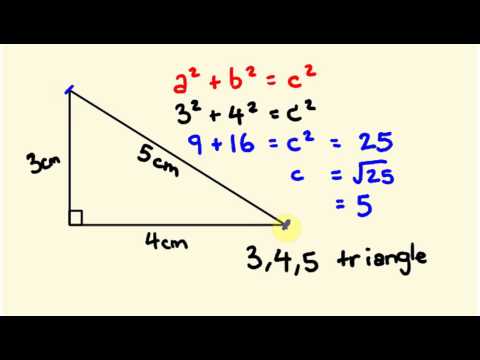
Pythagorus Theorum Math Lesson 3 4 5 Triangle Youtube
What Is The Radius Of The Incircle Of The 3 4 5 Right Triangle Quora

3 4 5 Triangle Angles Sides How To Solve Full Lesson

3 4 5 Right Triangles Explanation Examples
Solution Find The Interior Angles Of The Triangle With The Given Vertices 1 2 3 4 2 5
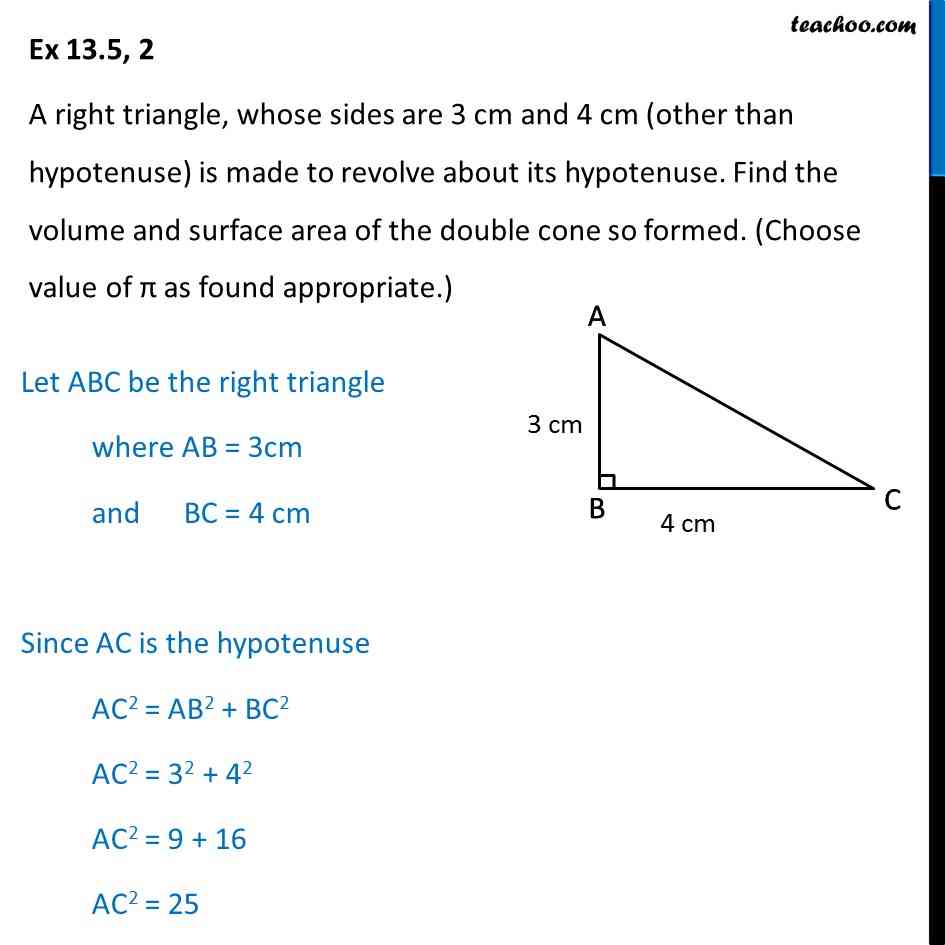
Ex 13 5 2 Optional A Right Triangle Sides 3 Cm 4 Cm Is Made To
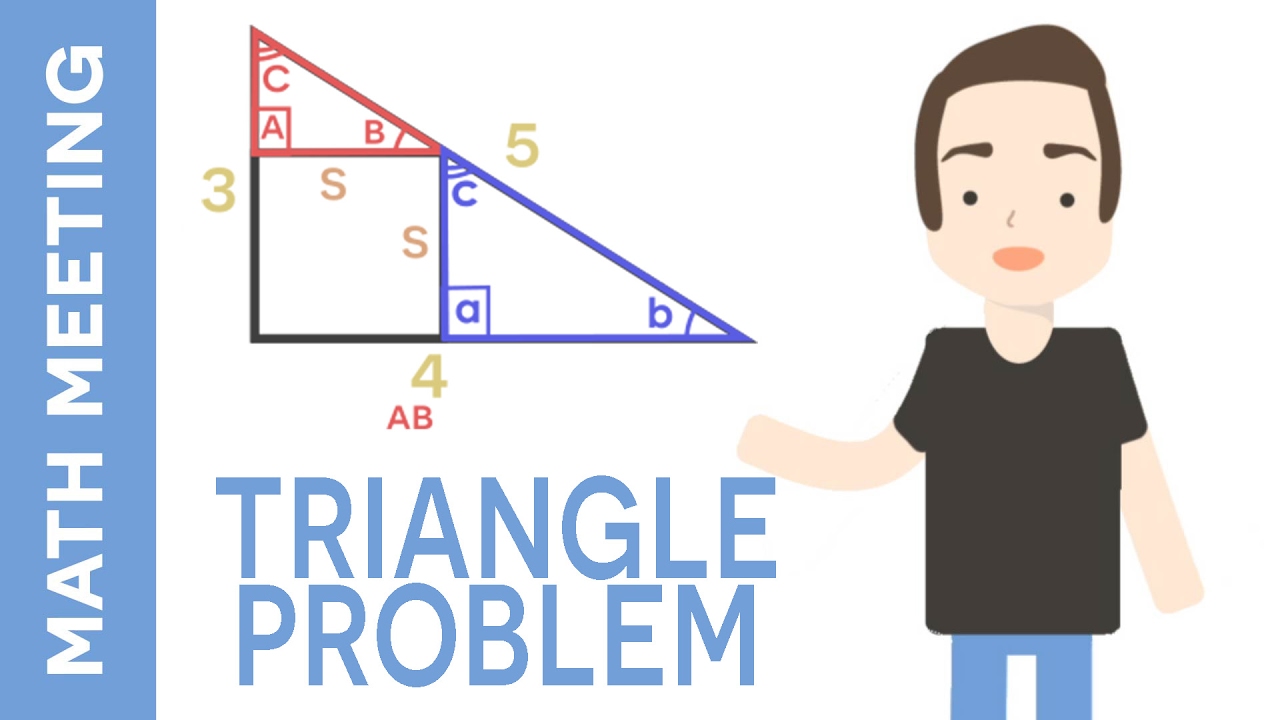
3 4 5 Right Triangles Explanation Examples

Illustrative Mathematics

Math Forum Ask Dr Math
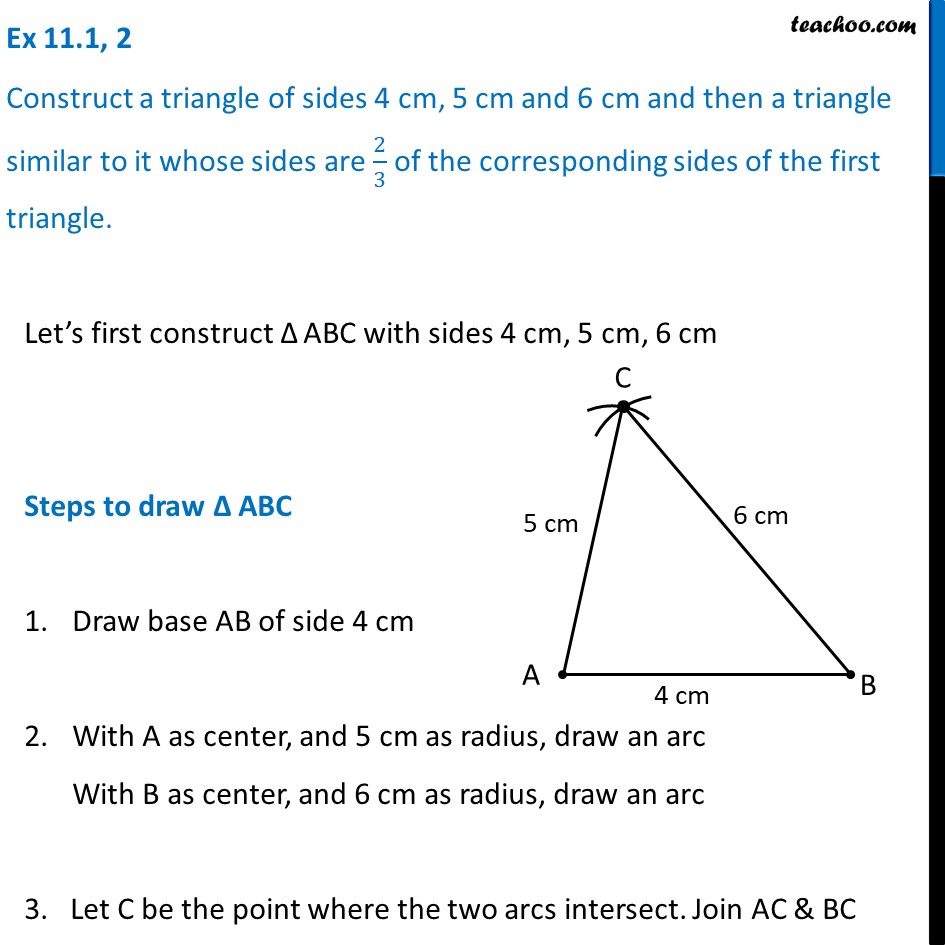
Ex 11 1 2 Construct A Triangle Of Sides 4 Cm 5 Cm And 6 Cm

A 12 Cm Wire Is Given A Shape Of A Right Angled Triangle A B C Having Sides 3cm 4 Cm And 5 Cm As Shown In The Figure The Resistance

The 3 4 5 Right Triangle Is A Commonly Used Right Triangle See Figure Use The Inverse Sine Function To Determine The Measure Of The Angle Opposite The Side Of Length 3 Express Your
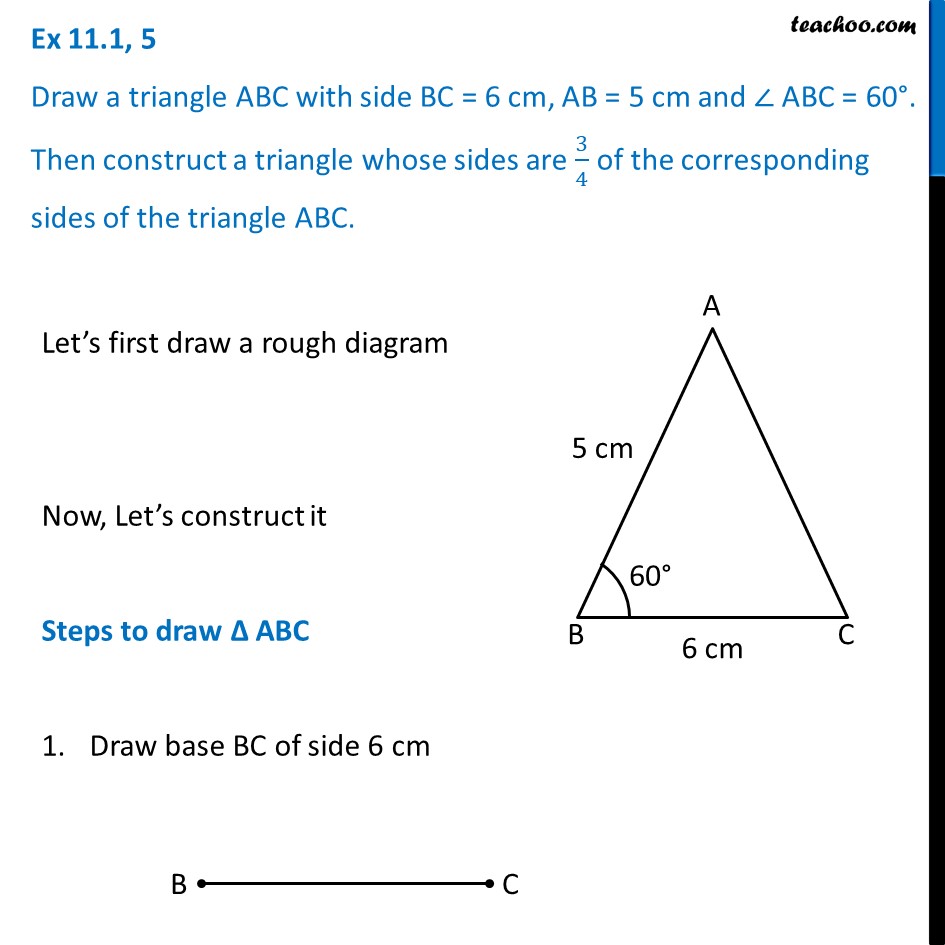
Ex 11 1 5 Draw A Triangle Abc With Side 6 Cm Ab 5 Cm Angle
Special Angle Values 30 60 90 And 45 45 90 Triangles Purplemath
Cosecant Cosecant Function Csc
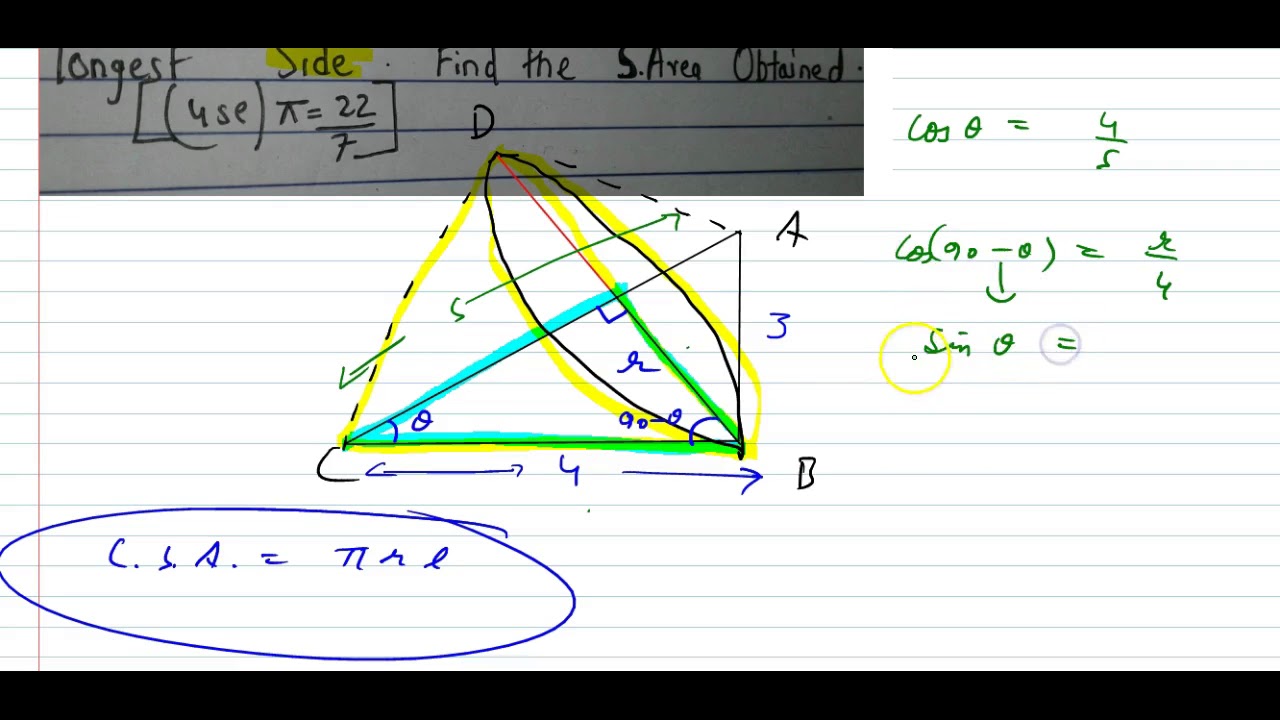
A Right Angled Triangle Whose Sides Are 3 Cm 4 Cm And 5 Cm Is Revolved Around The Longest Side Youtube

3 4 5 Triangle

The Sides Of A Triangle Are In The Ratio 3 4 5 The Measure Of The Largest Angle Of The Triangle Brainly In

Will Martinez Wmbassboy5 On Pinterest

3 4 5 Triangle Angles Sides How To Solve Full Lesson

What Is The Square S Side Length Mind Your Decisions

Numbers Angles Right Angles
Math Scene Trigonometry Sine Cosine And Tangent Lesson 1
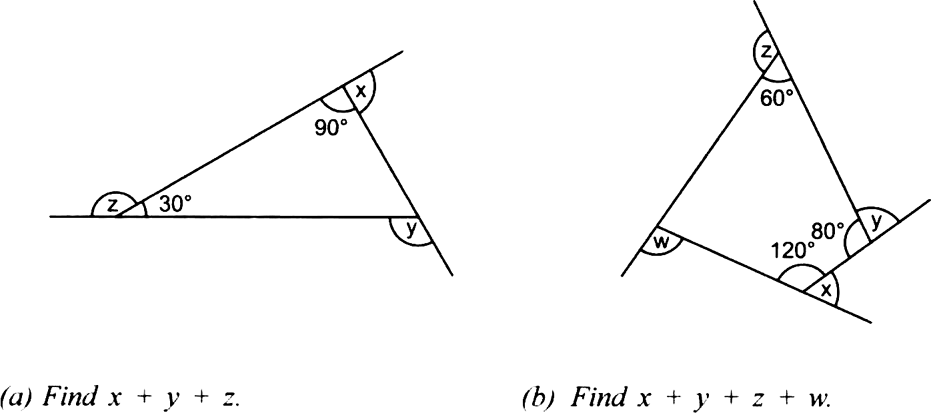
Examine The Table Each Figure Is Divided Into Triangles And The Sum Of The Angles Deduced From That Figure Side 3 4 5 6 Angle Sum 180 2 X 180 4
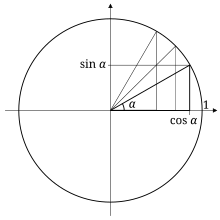
Special Right Triangle Wikipedia
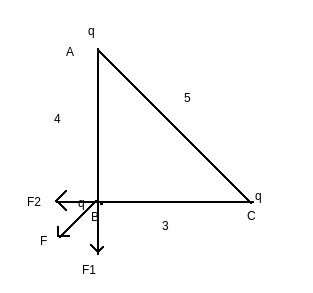
Three Equal Charges Which Has A Magnitude Of 2a 10 6 Coulomb Is Placed At The Corners Of Right Angled Triangle Of Sides 3 4 5 Find The Force On The Charge At Right Angle Corner

What Is The Radius Of The Incircle Of The 3 4 5 Right Triangle Quora
Gmat Geometry Everything You Need To Know
Special Right Triangle 30 60 45 45 37 53 Elearning
Solved The 3 4 5 Right Triangle Is A Commonly Used Right Chegg Com

Practice Worksheet 7 1

Pythagorean Triple 3 4 5 Docx
The Pythagorean Theorem
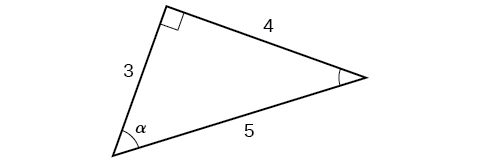
Right Triangle Trigonometry Algebra And Trigonometry

If The Sides Of Triangle Are In The Ratio 3 4 5 Prove That It Is Right Angled Triangle Brainly In

Unit 3 Section 1 Pythagoras Theorem
Construct A Triangle Similar To A Xyz With Its Sides Equal To 3 4 Th Of The Corresponding Sides Of Xyz Studyrankersonline

4 Setting Out Right Angles And Perpendicular Lines
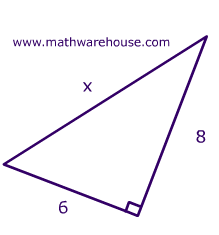
Find The Side Length Of A Right Triangle
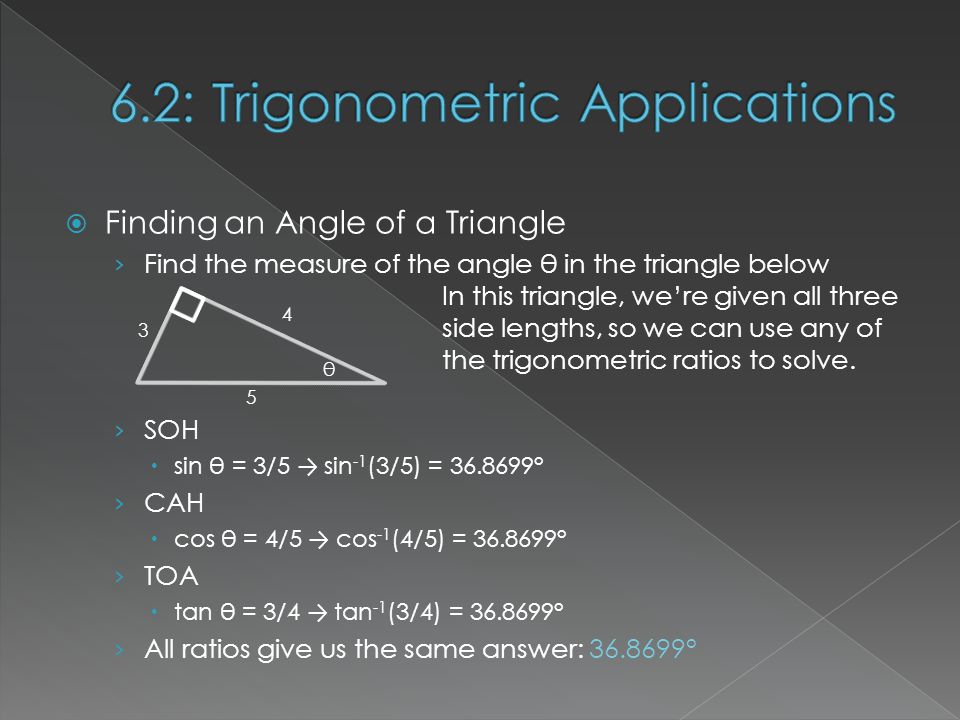
Old Stuff Will Be Used In This Section Triangle Sum Theorem The Sum Of The Measures Of The Angles In A Triangle Is 180 Pythagorean Theorem Ppt Download

Getting Square With The 3 4 5 Triangle
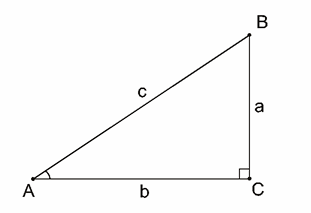
Math Scene Trigonometry Sine Cosine And Tangent Lesson 1

Math Guy S Weekly Comic And Puzzles 14 12 07

3 4 5 Triangle Definition Math Open Reference
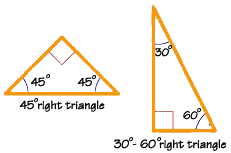
Relations And Sizes Right Triangle Facts In Depth
An Image That Shows A Right Triangle A Triangle In Which One Of The Internal Angle Is 90 Degrees A Polygon That Contains Three Edges And Three Sides Stock Vector Image
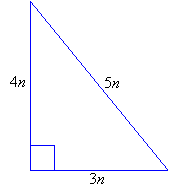
3 4 5 Right Triangles Worked Solutions Examples Videos
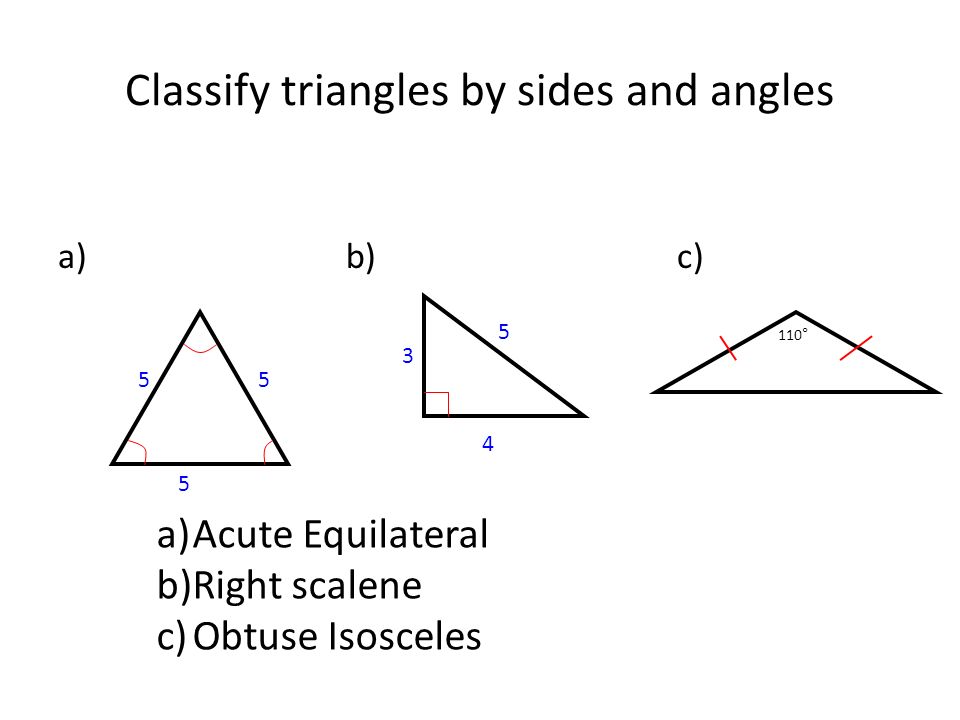
Triangle And Quadrilateral Review Acute Triangle An Acute Triangle Has Three Acute Angles Ppt Download
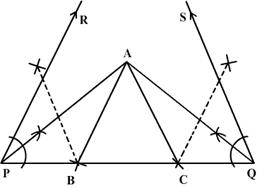
Construct A Triangle Abc Having Perimeter 12 Cm And Angles In The Ratio 3 4 5 Mathematics Topperlearning Com Gyq8yb6tt
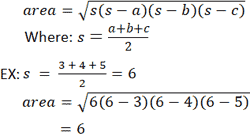
Triangle Calculator

3 4 5 Triangle Definition Math Open Reference
Is A 3 4 5 Triangle Also A 30 60 90 Triangle Quora

Triangle Wikipedia

The Sides Of A Triangle Are In The Ratio 3 4 5 If It S Perimeter Is 36 Cm Area Of Triangle Is Brainly In



